Monica Kochar
Tangram, now considered a great puzzle, is actually an ancient art. The story goes that the legendary Chinaman ‘Tan’ first conceived it. In Chinese this puzzle is called ch’ich’iaot’u—an ‘ingenious puzzle figure of seven pieces’.To play you simply need to rearrange seven pieces, each called a ‘tan’ to exactly reproduce a given image. While there is no evidence to corroborate this story it is known that it was a popular recreation in China near the start of the 19th century. This was followed by a craze in America, Europe, and Asia where it was one of the most popular games of the 19th century.
The pieces are: a small square, two small congruent triangles, two large congruent triangles, a medium-sized triangle and a parallelogram.
They look like:
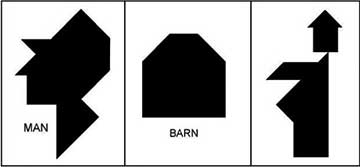
The objective is to create images using these seven pieces.
For example:
As you can see, these images are either geometric in nature or patterned after a familiar object. Thousands of such images can be made thus making the tangram a very interesting and challenging puzzle.
How can we make the tangram pieces?
Making the seven pieces is easy! There are two possible ways, my favourite way being the second way.
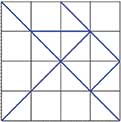
- Take a thick 4 x 4 square shaped paper and draw blue lines as shown. Cut along the blue lines carefully to get the seven pieces.
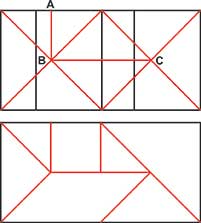
- Take a matchbox and flatten it to get a rectangle.
What you have in the above picture are four rectangles with width in the ratio of 1:2 as shown below.
Draw red lines as shown on the rectangle obtained from the matchbox.
As you cut along the red lines you get the required seven tans!
Why use the tangram in a classroom
There are many reasons why one should consider using the tangram in the classroom.
- A tangram is an excellent brain exercise in spatial coordination.
- It helps develop the capacity to discern the whole from its randomly scattered parts. As you stare at the visual pattern and think about it using the “mind’s eye”, it boosts the visual-spatial skills.
- It helps classify shapes, be positive about geometry and also understand geometry vocabulary.
- It is also just a fun puzzle to solve!
The tangram in the math classroom
There are many ways that the tangram can be used in the class.
Geometry
The seven tans can be used to make various geometrical shapes.
- Divide the class into groups of four and let each group make a set of tangram pieces.
- Check if all groups have the seven pieces of tans.
- Ask the students to assemble the seven pieces to make the following shapes:
a. A quadrilateral with four right angles and equal opposite sides.
b. A quadrilateral with two sets of parallel sides and no right angle.
c. A quadrilateral with one set of parallel sides and no right angle.
d. A quadrilateral with four right angles and all equal sides.
Giving exercises like these will help students connect shapes with their properties. - Once they complete the task set, ask them to compare their results with the answers given here:
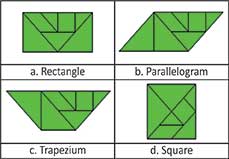
- See if anyone has used a different way to assemble the pieces. Ask them to demonstrate it.
- Ask the students to trace the shapes assembled on plain paper and check if the shapes are accurately designed to form a rectangle, a parallelogram, a trapezium, and a square.
- Ask the students to reason as to how they are sure that their answers are correct. Their answers should demonstrate their knowledge of the properties of the shapes.
Area investigation
- Divide the class into groups of four and let each group make a set of tangram pieces.
- Ask each group to organize the tangram pieces to get a square.
They should get: - Investigate the area relations in the obtained square. This will test their skills of fractions also.
- Make a square with two small congruent triangles. What is the area of this square?
- Make a triangle with two small congruent triangles. What is the area of this triangle?
- Make a square with two large congruent triangles. What is the area of this square?
Investigate: If the area of the big square is 1 sq.m, what is the area of each of the seven tans? If students are not comfortable with 1 sq.m, take the area as some multiple of 2,4,8,16 (16/32/…) and then ask again for 1 sq.m.
Answer:
The combination of the 2 large triangles represents 1/2 of the whole square. The area of each large triangle hence is 1/4. The medium triangle, square, and parallelogram each is 1/8 of the whole area of square. Each small triangle is 1/16 of the whole area of the square.
At each step, let the students justify their answers with reasoning. “Conversations to give reasoning” helps develop the mathematical mind.
Puzzles
Let the students create the following shapes using the tangram pieces.
(Source http://www.funorama.com/tangram-challenges-1.html)
Storytelling
This is an interesting idea I found on a website. (Check http://www.logicville.com/puzTS-A1.htm for the complete story). Give students a story and ask them to make the tangram puzzle asked for to match the story.
It starts as:
One upon a time, there was a crow.
(Puzzle for students)
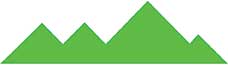
The crow flew over a mountain.
(Puzzle for students)
RESOURCES FOR MORE
Endless material is given on the Internet on how tangrams can turn in to a fun educational aid. Some of the good sites that you can refer to have been listed within the article. You could also simply go to a search engine and look for “tangram worksheets” for more ideas.
So the next time you think your students are not ready for a math class, simply pull out your tangrams and see the atmosphere change.
The author has been a teacher for 19 years and is on a sabbatical from ‘schooling’. She is now a freelance math curriculum developer and is experimenting with strategies to make math accessible to all. She can be reached at reachmonica@gmail.com and has a blog titled http://humanemaths.blogspot.in.